Why bodies have "mass" and "inertia"
     The blueshifted photons are redshifted when absorbed and reemitted, and the redshifted photons are blueshifted when absorbed and reemitted. For θ=0°, a blueshifted photon with energy ep=2 is redshifted by a factor of (ca−va) or .4 when absorbed and by a factor of ca/(ca+va) or .625 when reemitted, for a combined factor of (.4·.625) or .250. Therefore the energy of the reemitted photon is (2·.25) or .5 ep. This is the same as the energy for photons emitted from the center toward 180°. Similarly, the photons reemitted at 30° have the same energy as the photons emitted from the center toward 210°.

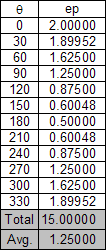
     The table shows that for every blueshifted photon emitted at the center or emitted at the shell surface there is a redshifted photon emitted in the opposite direction, and that their combined energy is 2.5 ep and their average energy is 1.25 ep. (Note: There are more blueshifted photons than redshifted photons in the body because they move more slowly through the body.) The increase in average energy emitted is equal to the work done to accelerate the body to va=.6 ca and increase the body's mass/energy by a factor of 1/rv or 1.25, as will be seen shortly. This increase in mass/energy is in exact agreement with the predictions of orthodox theory and experimental evidence. It is consistent with the following equation where m is a body's mass and m_o is the body's at-rest mass.


     We have seen that the pattern of energy quanta in a body changes when the body's velocity through the qm changes. After acceleration to va=.6 ca, much more of the body's internal energy is moving in the +x direction than in the −x direction. Changing the internal energy in a body requires a force and work, whether the internal energy is increased or decreased. In the quantum medium view, this is the source of a body's "inertia."